Using Correlation Coefficients in Research Papers

Academics have many options when it comes to the research they want to complete. A great option, especially for students, is correlational research. As you'll soon see, this type of analysis is fantastic for conducting quick research on a small or non-existent budget.

In this article, you'll learn about correlational research and correlation coefficients. You will be guided through the steps of gathering, analyzing, and reporting correlational data in your research papers. By the end, you should have a solid understanding of how to find correlation coefficients and use them to present your research findings.

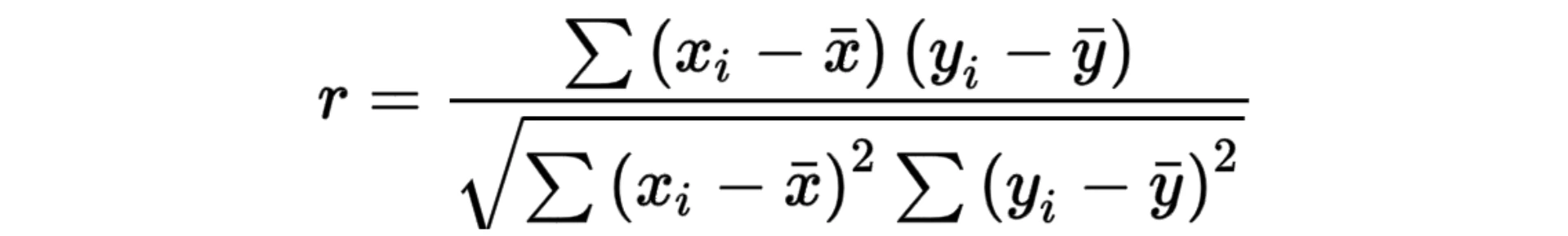
Introduction to Correlation Coefficients

A correlation measures the linear relationship between two variables. The correlation coefficient describes the strength and direction of the relationship between those variables. The correlation coefficient ranges from -1 to +1, where 0 describes no relationship between the variables, and an absolute value of 1 describes a perfect relationship between the variables.

The purpose of correlational research is to pinpoint whether there is some sort of linear relationship between a couple of variables. For example, you may want to know whether the amount of time a student spends studying correlates with the grade they receive. By surveying the students in one class, you could gather data on the number of hours they study per week, along with the final grade they received for that class. Plugging that data into a correlation coefficient formula, you would receive a value ranging from -1 to +1. That value would tell you if grades increase as study hours increase, if they decrease as study hours increase, or if the grades did not have a discernable pattern based on hours studied.

However, it's important to understand that correlation is not equal to causation. If you found, using correlational research, that grades improved as students spent more time studying, you wouldn't be able to conclude that studying more causes students to receive better grades. As you will see later, there can be other explanations for the results you find.

How Is Correlational Research Useful?

If you can't determine causation with correlational research, you may wonder what the point of it is. Why not just conduct experimental research instead? Here are the main reasons someone would consider correlational research:

• It's much quicker than experimental research. With correlational research, you can gather data from natural (non-experimental) settings in a relatively short time.
• It's generally cheaper than experimental research. Correlational research normally involves less time and resources than experimental research, making it a cheaper and easier option.

When Would You Use Correlational Research?

There are a few instances where correlational research is beneficial, even more so than experimental research:

• Investigating non-causal relationships. You won't always expect a causal relationship between two variables, but it can still be helpful to know if they correlate.
• Supporting causal relationship theories. It can sometimes be too expensive, impractical, or even unethical to run experiments that would determine a causal relationship between variables. In those cases, a strong correlational relationship can support the theory of a causal relationship.
• Testing new measurement tools. If a correlational relationship between variables is already well-known, you can conduct correlational research on those variables using new measurement tools to measure their validity and reliability.

Defining Correlation Coefficients

The value of the correlation coefficient details how strong the relationship between those variables is. A value of -1 or +1 is a perfect correlation, meaning that the relationship between the variables is perfectly linear. The closer the value gets to 0, the less linear the relationship is. Whether the value is positive or negative is also important, as that tells you the direction of the relationship. A negative relationship means that if one variable increases, the other variable decreases. A positive relationship means that if one variable increases, the other variable increases as well.

There are multiple different correlation coefficients you can use depending on the data that you've gathered. The correlation coefficient formula also differs depending on the coefficient you're looking for.

Types of Correlation Coefficients

• Pearson's r: The relationship between two continuous, random variables that are both normally distributed. Data must meet these criteria to use Pearson’s accurately.
• Spearman's rho: The relationship between two continuous or ordinal variables. These variables do not need to be normally distributed to use Spearman's rho, and this correlation coefficient is commonly used when the criteria for Pearson's are not met. This coefficient is based on the ranking of the data and not their actual values.
• Kendall's tau: This is an extension of Spearman's rho. You would use this coefficient when using a small dataset and one rank is repeated too many times.
• Phi Coefficient: The strength of the relationship between two categorical variables in a 2x2 contingency table.
• Cramer's V Correlation: The strength of the relationship between two categorical variables in contingency tables larger than 2x2.

Collecting Correlational Data

Just like experimental research, correlational research uses quantitative research methods. The difference is that the variables in correlational research are only observed and not manipulated as they would be in experimental research. There are a few different ways to collect correlational data:

1. Surveys: You can use questionnaires to quickly collect data from your population of interest. This can be done in person, but it can more easily be done online, by mail, or by phone. 
2. Observation: This type of data collection involves observing behavior or phenomena in a natural environment. This often involves recording, counting, and describing the environment, events, and actions you're recording.
3. Secondary sources: Not all data needs to be original, and you can find sets of data that have already been collected for several different purposes. This is the fastest and most inexpensive way to collect data, but it can also be unreliable. As you didn't collect it yourself, you don't have any control over the reliability and validity of the data.

Analyzing Correlational Data

Analyzing correlational data begins with plotting the data and calculating the correlation coefficient. As previously mentioned, the correlation coefficient will provide you with a value that represents the strength and direction of the relationship. Plotting the data on the graph will provide you with a visual representation of that relationship.

For determining the strength of the correlation, there are some general guidelines on correlation coefficient interpretations.

There are a couple of caveats to keep in mind when analyzing and interpreting the results of your correlational analysis. The first is that values near 0 don't necessarily mean that there is no relationship at all between the variables. It only means that there's no linear relationship. However, there could be some other relationship, such as a quadratic relationship. Graphing the data before performing an analysis will allow you to see what the relationship looks like.

The other is that, once again, correlation is not causation. When you discover a correlational relationship, there could be multiple reasons for that result that aren't accounted for in research. 

One issue is what's known as the directionality problem. Let's think about the studying and grades example, and we find that as students study more, their grades increase. You could assume that studying more results in better grades, but you could equally assume that getting better grades results in more studying.

Another possible issue is a third variable that wasn't considered in the research. With the studying and grades example, it could be another variable that increases both studying and grades, and that those two don't affect each other in any way. It's entirely possible that students who sleep more tend to study more and get better grades.

Present Both Good Research and Writing

Correlational research is a great option for students, and academics in general, to study relationships between variables. There are a variety of ways to gather, analyze, and interpret correlational data. This information should provide you with a solid foundation to get started on your own correlational research. For more information on conducting research and writing your research papers, take a look at our resources page. When you're ready for rewriting services or proofreading services, our PhD writers and editors are available to help.